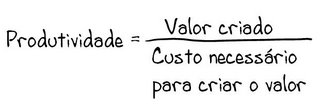
Bent Flyvbjerg no livro "How Big Things Get Done" refere que por vezes os projectos saem furados não por causa da execução, mas por causa das previsões irrealistas com que foram baseados:
“When delivery fails, efforts to figure out why tend to focus exclusively on delivery. That’s understandable, but it’s a mistake, because the root cause of why delivery fails often lies outside delivery, in forecasting, years before delivery was even begun.”
Uma das formas de evitar estas previsões irrealistas passa por usar informação de projectos anteriores (o título do capítulo é "SO YOU THINK YOUR PROJECT IS UNIQUE?" e o subtítulo é "Think again. Understanding that your project is "one of those" is key to getting your forecasts right and managing your risks.""
Muitos projectos seguem uma distribuição normal.
"But even with a project as simple as a kitchen renovation, the number of possible surprises, each unlikely, is long. Many small probabilities added together equal a large probability that at least some of those nasty surprises will actually come to pass. Your forecast did not account for that."
No entanto, os projectos grandes podem seguir um outro tipo de distribuição:
"There is, however, a big, fat-tailed caveat on all this. Imagine you have a graph with the costs of one thousand kitchen renovations that takes the shape of a classic bell curve—with most projects clustered around the mean in the middle, very few projects on the far right or far left, and even the most extreme data points not far removed from the mean.
...
But as noted in chapter 1, my analysis revealed that only a minority of the many project types in my database are “normally” distributed. The rest—from the Olympic Games to IT projects to nuclear power plants and big dams—have more extreme outcomes in the tails of their distributions. With these fat-tailed distributions, the mean is not representative of the distribution and therefore is not a good estimator for forecasts. For the most fat-tailed distributions, there isn’t even a stable mean that you can expect outcomes to cluster around because an even more extreme outcome can (and will) come along and push the mean further out, into the tail toward infinity. So instead of good old regression to the mean, you get what I call “regression to the tail.” In that situation, relying on the mean and assuming that your result will be close to it is a dangerous mistake.
...
If you face a fat-tailed distribution, shift your mindset from forecasting a single outcome (“The project will cost X”) to forecasting risk (“The project is X percent likely to cost more than Y”), using the full range of the distribution.
...
Contingencies might have to be 300, 400, or 500 percent over the average cost—or 700 percent, as we saw for the Montreal Olympics. That’s prohibitive. Providing such contingencies would not be budgeting; it would be blowing up the budget. So what can you do about the tail? Cut it off. You can do that with risk mitigation. I call it "black swan management".
...
Some tails are simple to cut. Tsunamis are fat-tailed, but if you build well inland or erect a high enough seawall, you eliminate the threat. Earthquakes are also fat-tailed, but build to an earthquake-proof standard, as we did with the schools in Nepal, and you are covered. Other tails require a combination of measures; for a pandemic, for instance, a blend of masks, tests, vaccines, quarantines, and lockdowns to prevent infections from running wild. That’s black swan management.
...
The critical next step is to stop thinking of black swans the way most people do. They are not bolt-from-the-blue freak accidents that are impossible to understand or prevent. They can be studied. And mitigated."
Um exemplo da mitigação apresentado é: construção de uma linha ferroviária em Inglaterra. Qual o motivo mais comum para paragem de uma obra? Achados arqueológicos! Mitigação: Contratar arqueólogos para estarem de prevenção para intervirem e reduzirem tempo de paragem.